NOTES
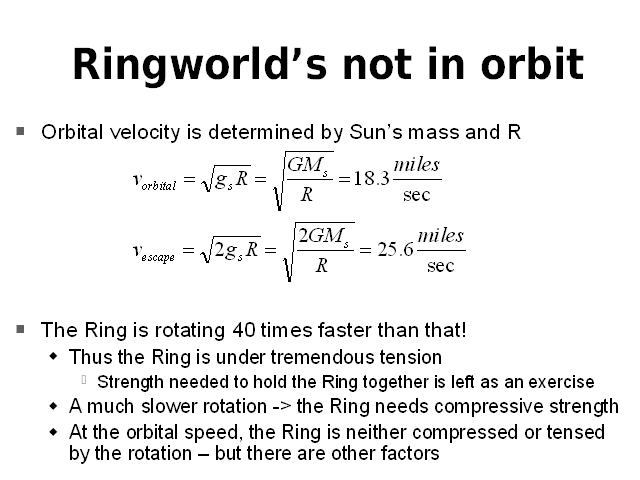
The Ringworld is not in orbit.
Orbital speed, v, for a circular orbit at any given radius, R, is the square root of GM/R where G is the universal gravitational constant (6.67300 × 10^(-11) m^3 kg^(-1) s^(-2)) and M is the mass of the Sun. For the Ring this velocity is about 18 miles per second; since the velocity of the ring is about 40 times that, the Ring is in tension (meaning that absent the structural strength of the Ring, each part of it would pull away from the rest of it (and away from the Sun)). If the velocity of the Ring is less than orbital velocity, then the Ring is in compression, meaning that absent the structural strength of the Ring, each part of it would be pressed further together (and further into the Sun of course). If the velocity of the Ring is exactly orbital speed, then each portion of the Ring , under neither compression nor tension – even if the Ring was made of wood or butter, it would retain its shape, with respect to the forces due to the Sun and its rotation anyway (or would it – see later slides).
Exercise for the student of materials - how strong must the Ringworld’s material be?
