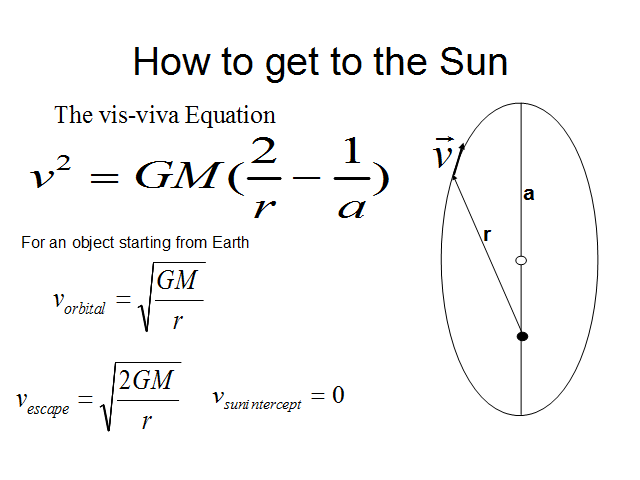
NOTES:
To calculate the amount of thrust needed, use the vis-viva equation - it's one of the handiest equations for dealing with orbital mechanics in a science fictional environment. The total velocity of an object at any point in its orbit is equal to a constant times the difference between twice the reciprocal of the current distance from the primary and the reciprocal of the average distance from the primary. What this gives you (among other things) is the delta-v for transfer orbits. If you want to travel from earth to Mars, simply calculate the current orbital velocity of earth, and then the orbital velocity of an object currently at earth’s radius but with a maximum distance that just reaches Mars' orbit. That gives you the dV to apply. Once you reach Mars the same equation gives the dV that will put the spacecraft in the same orbit as Mars.
What makes this equation particularly useful is that you can pick your units for your convenience. When I was taking courses I used units of astronomical units for r and a, and units of miles per second for velocity whenever possible, so that for calculations in the solar system I could determine GM by taking the earth case - all I needed to remember was the length of the year and the size of earth's orbit and I could determine the earth's orbital velocity, and since the distance term in my units was (2-1), GM was easy to solve for and easy to use for any subsequent solar system problem without the pain of memorizing G or M.
So to get to the Sun from the Earth, you need to thrust enough to reduce your velocity from orbital velocity, to the velocity of an orbit that strikes the Sun; this would be an orbit with a semi-major axis of 0.5 astronomical units; that velocity is 0. So the delta-V to get to the Sun is 30 km/s, while the delta-V to escape completely from the solar system is only 12.5 km/s.
