NOTES:
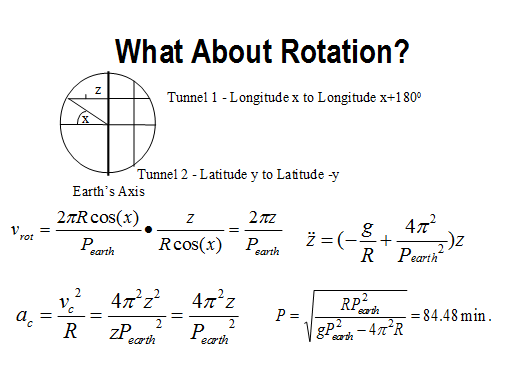
Now some of you are probably wondering about the effect of the Earth's rotation on this method of travel. There is one, but it's not as bad as it might seem. Tunnel 1 runs parallel to the earth's rotational axis, so the any acceleration due to the rotation is always perpendicular to the motion of Killeen's body, and so has no effect on that motion (as long as we assume the tunnel is completely frictionless). Tunnel 2 goes through the earth's rotational axis, so the acceleration due to the earth's rotation is always outward along the path of the tunnel. This requires some further analysis. The rotational velocity at the surface of the earth at latitude x is given by the distance of the surface from the axis and the period of the earth's rotation. The rotational velocity of a point in the tunnel is linearly related to that and to the distance of the object from the earth's axis. Combining these two factors we get a rotational velocity in terms of distance along the tunnel and the period of the earth's rotation. The acceleration due to this rotational velocity is given by this familiar equation, giving us the outward acceleration due to the earth's rotation (frictionless walls are really needed here, because the object would touch the walls throughout its trip). Combining this with the gravitational acceleration derived earlier, our differential equation is still simple and the solution is still harmonic motion, just with a different period. The new period of Killeen's oscillation through the earth is only 8 seconds longer than it was before. So, a trip that goes to an opposite latitude while staying at a given longitude is acceptable, a trip that goes to an opposite longitude at a given latitude is also acceptable, and in fact, any trip with a constant latitude (even if it did not go through the axis) would also be acceptable and have the same period as derived above. What's not acceptable? Well, any trip that changes latitude, but doesn't go to an opposite latitude would cause a rotational acceleration that was always in the same direction - Killeen would rocket out of the tunnel or perhaps get stuck part way through. I calculated one specific example - imagine a tunnel going from the North pole to the equator. It turns out that the final velocity of Killeen as he left the tunnel at the equator would be about 3 tenths of a mile per second - pretty fast. If the earth rotated as fast as Hal Clement's Mesklin (Mission of Gravity) the rate of egress would be a lot faster, but I wouldn't be justified in assuming a spherical Earth any more.
