NOTES
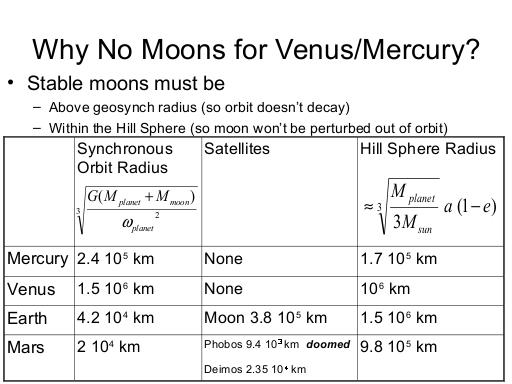
With that as background it's easy to figure out why it's not a coincidence that Mercury and Venus have no moons. As we've previously discussed, moons below the geosynch radius will have orbits that tend to decay, leading to an eventual collision with the planet. It's also true that each planet has a volume around which it will not lose a moon to the Sun - this is called the Hill Sphere (http://en.wikipedia.org/wiki/Hill_sphere), and depends on the planet's mass, the mass of the Sun, the distance from the planet to the Sun, and the eccentricity of the planet's orbit. So to have a long-lasting moon, which is neither perturbed out of orbit nor decays in orbit until crashing into the planet or being disrupted into a ring, the moon must be within the Hill Sphere (preferably well within it) and outside the geosynch radius - and for Mercury and Venus there is no such place; the Hill sphere is inside the geosynch radius.
Earth is better off - it has a large Hill Sphere because of its mass and distance from the Sun, and it has a low synchronous orbit radius because of its rotational speed. The Moon is well within the Earth's Hill Sphere and even when the Earth and moon become fully tidally locked that will remain true, so the Moon can remain orbiting the Earth for the rest of the life of the Solar System.
Mars is an interesting case - it has room for a moon to be outside the geosynchronous radius but inside the Hill Sphere, but only one of its moons is so fortunate. Phobos will eventually collide with Mars, or be disrupted and form a ring of particles around the planet.
After the convention I realized that it's possible to determine if a planet can have a long-lasting moon without going to the trouble of calculating the synchronous orbit and the Hill Sphere radius. A long-lasting moon is possible if the synchronous orbit radius is smaller than the Hill Sphere radius, which, after some algebra amounts to P_orbit (1-e)^ □(64&3/2)(1/√3) (1+M_p/M_m)/(1+M_m/M_p)>P_rotation where Porbit is the orbital period of the planet and Protation is the rotational period of the planet. In other words, unless a planet's year is significantly longer than its day, no long-lasting moon is possible.
