NOTES
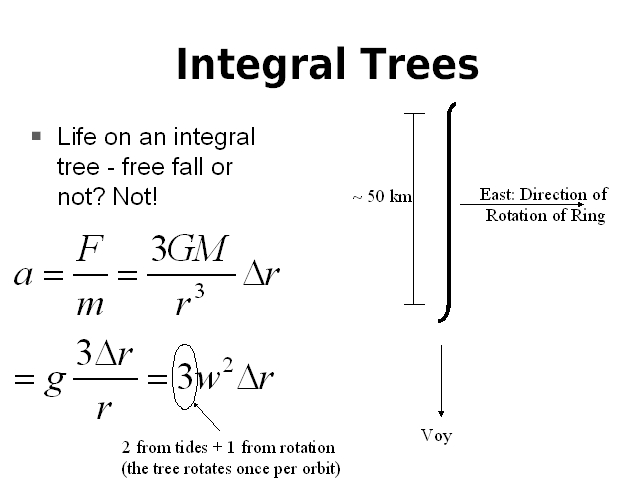
One of the life forms common in the Ring is the Integral tree. It’s pretty big - people actually live on it. Now when people are simply in the Smoke Ring they’re in free fall, but what if they live on a tree? It turns out that tides give an equivalent to gravity.
The gravity of the neutron star is so great that the ends of the tree should be in significantly different orbits, but instead they’re constrained to orbit together. Therefore anything attached to the upper end of the tree is moving too fast for its orbit and thus feels an acceleration outward. Meanwhile, anything attached to the lower end is moving too slowly for its orbit and is accelerated inward towards Voy. The middle of the tree remains in free fall (otherwise the whole tree would accelerate into Voy or out of the Ring) - it’s in the right orbit after all. Another way to think about it is that the whole tree is being swung around Voy, which should result in a centrifugal force outward on any object on the tree, but the gravitational force of Voy cancels out the centrifugal force exactly at the center of the tree, too much on the inner tuft and not enough at the outer tuft.
The equation for the acceleration experienced by something attached to the tree is remarkably simple - it’s just 3 times square of the rotation rate of the tree around Voy times the distance of the object from the center of the tree. In other words, it’s just the force that would be felt if Voy didn’t exist, and the tree were being rotated around its center at the rate omega, plus the differential force due to change in Voy’s gravity over the length of the tree (Thanks to Peter Taylor for pointing out a factor-of-3 error in an earlier version of this talk).
Unfortunately while the book reports a maximum force of about 1/5 g at the ends of a tree, I get a force of almost 30 gees - probably because of the same miscalculation that caused the error in the period of objects in the Smoke Ring. However, that problem is easily resolved - just make the tree shorter. By the way, the same tidal force that creates pseudo-gravity for objects attached to the tree makes the orientation of integral trees very stable - each end of the tree is being pulled so that it lines up with the radial vector. If the tree ever got out of that orientation, it would oscillate a bit, but then settle down due to the damping effect of air resistance. I should note that man-made satellites orbiting the earth sometimes use tidal stabilization to remain oriented with one axis pointing towards the earth.
